IJCRR - 10(14), July, 2018
Pages: 22-29
Date of Publication: 18-Jul-2018
Print Article
Download XML Download PDF
Mathematical Model on two layer Blood Flow in capillaries with context to Diabetes
Author: Tarunika Sharma, Rashi Khubnani, Harish Chandra
Category: Life Sciences
Abstract:In this paper the review of mathematical modeling of two phase blood flow in capillary is done with disease Diabetes .There are two phase of blood flow, one is RBC and other one is plasma. By the Fahreaus-Lindqvist influence the blood flow in two separated layers as transient from side to side capillaries, in which one layer is Newtonian and other is consider as Non-Newtonian layer. Further Non-Newtonian power law model is applied to bio fluid mechanical system. We have collected an observation data for patient having Diabetes. On the whole arrangement is in tensorial form and key method adapted is investigative as well as arithmetical. Later the graphical representation is also given for the data used here.
Keywords: Blood Pressure, Hematocrit, Renal circulation, Glomerular capillary, Diabetes, Per tubular capillary, Circulatory system
Full Text:
INTRODUCTION
Composition of Kidney: Kidneys are bean shaped organs. Kidney has an outer fibrous renal capsule and supported by adipose tissue. There are two main parts, inner medulla and cortex. External cortex is reddish brown, where fluid is clean from blood. Inner medulla is paler and prepared by conical formed sections call renal pyramids. [1][2] The medial edge of the kidney is called the hilus and is the part where the renal blood vessels depart and come in the kidney [3][4][5][6]. The main purpose of kidneys is giving out the blood and takes away waste and surplus water through the urine. Both kidneys are comprised of million filter systems named nephrons. Each nephron filters little quantity of blood. It has a filter that is glomerulus, and a tubule. Glomerulus allow fluid and waste items bypass through it, although, it prevent blood cells and big molecules, typically proteins, from passing. The clean fluid then passes from tubule, which sends out essential minerals reverse to the blood stream and eliminates waste. [7][8]
Blood contribute to kidney: In the stomach, the renal artery stem from abdominal aorta lower to the bigger mesenteric artery and enlarge crossways in the direction of the kidneys. Just earlier to reach the kidney, all renal artery separates keen on five segmental artery, which supply blood to the variety of regions of kidney. Every segmental artery goes into the hilus of the kidney furthermore divides into quite a few interlobar arteries; get in front of the renal columns linking the renal pyramids and take blood in the direction of the peripheral of the kidney. At the link in the midst of the cortex and medulla, the interlobar arteries figure out arcuate arteries, which spin to go behind the contours of renal pyramids. Arcuate arteries form a number of branches, identified the same interlobular arteries, divide at right angles and enlarge the entire mode in the course of the renal cortex on the way to the outside of the kidney. In latent fully developed kidney get 1.2 to 1.3 l blood per minute. The Fick principle is appropriate to find renal blood flow [9] the renal blood flow is considered by dividing by one minus the hematocrit. [10]
2 Strain in renal vessel: Blood is a fluid tissue consists of approximately 55% of fluid plasma and 45% of cells. It consists of three major types of cells which are red blood cells, white blood cells and platelets. Blood plasma is made of 92% water and 8% of ions, Proteins, metabolites. The standard thickness of whole blood for a human is on 1060 kg/M3.[11] The force in glomerular capillary has been calculated directly in the rate and has been set up to be significantly lower than the predicted on the source of not direct dimension . When the mean systolic arterial force is 100 mmhg, then glomerular capillary force is about 45 mm hg. The pressure drop crossways the glomerulas is only 1 to 3 mmhg , but supplementary jump down occurs in the efferent arteriole such that pressure in the per tubular capillary as regards 8 mm hg. Pressure in renal vein is regarding 4 mm hg. [9]
Structures of renal capillary: Renal vascular model is extraordinary in this blood flows through two capillary beds, high pressure (glomerular) and low pressure (per tubular), associated in sequence. Blood enters the kidney by means of the renal artery and, following a chain of divisions, arrives at the glomerulus. Glomerular capillaries have to first go by afferent arteriole, where blood passes all the way through a second arteriole, the efferent arteriole. After that blood flows through the per tubular capillaries, which comprise the vasa recta that enlarge into the renal medulla, after this it accumulates in gradually larger venules and veins, and then goes out of the kidney through the renal vein. [6] [12][13]
Material and Methods
Disease (Diabetes): Diabetes is a disease in which the body does not correctly absorb food for make use of energy. The majority of the food we eat is converted into glucose, for our body to utilize for energy. The pancreas makes a hormone named insulin to assist glucose for entering the cells of body. Body having diabetes will some time doesn't make sufficient insulin or can't take up its own insulin as how it should. This effect sugars to put up in blood. So diabetes is also referred as sugar. [14][15]
Explanation of the problem: Capillaries are very thin as well as distant from the heart, so in this situation how the blood flow is possible in these vessels. This is done by Fahreaus-Lindqvist effect. As per this effect the blood flows in two alienated layers while transitory through capillaries. The plasma layer contains more or less no blood cells. The second layer is blood cells which hang in plasma on the alignment of the capillary. In this procedure the useful blood viscosity depends upon radius of the capillary. So the effective viscosity decreases, with the radius and thus the blood flow becomes probable.[6]
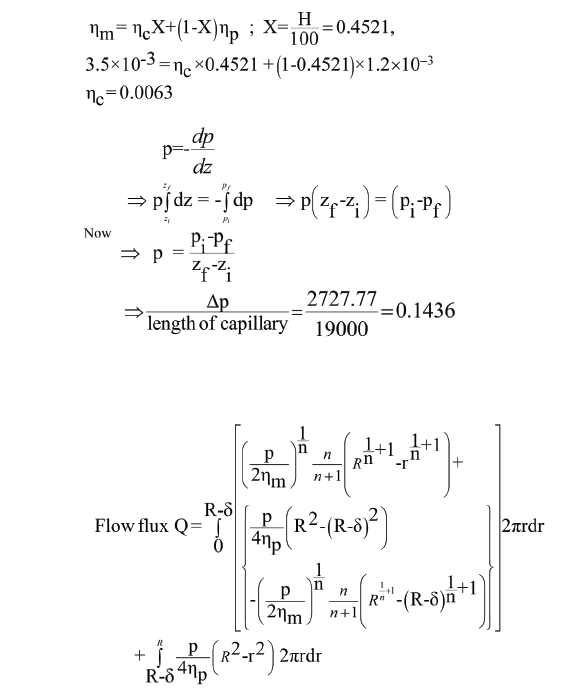
Model: Blood viscosity means thickness and tackiness of blood. Usually mature blood viscosity is 40/100, unit mill poise.[16] Blood is a dynamic organ in so far since it act as a non-Newtonian fluid, in the sense its viscosity changes with role of shear rate. Consider shear rate like velocity, when blood travels fast as in peak-systole, it is physically thinner; when it travels slowly during end-diastole, it is thicker and stickier, because red cells cumulate. This method is called as the shear-thinning, non-Newtonian character of whole blood. [17][18][19][20]. Here in the current paper we choose comprehensive 3- dimension orthogonal curvilinear co-ordinate arrangement, which is arranged as E3 known as three-dimensional Euclidean space. Here are some quantities related to moving blood in cylindrical vessels: blood velocity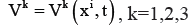 
blood pressure 
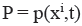and density 
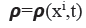where xi is co-ordinates of any random point in space and i-1,2,3.
Hematocrit: It is the relative amount of the quantity of red cells to the quantity of whole blood. Normal array for hematocrit is dissimilar among the sexes and is roughly 44% to 51% for men and 36% to 47% for women. The hematocrit (articulated as percentage) is usually as regards three times the hemoglobin (grams per deciliter). It is denoted by H.[6] [21][22][23][24]
Equation of Continuity: As there is no source or sink in the whole circuit of the human blood circulatory system, the heart behaves simply like a pumping station that is why the law of conservation of mass is applied to hemodynamic [25]. In view of the fact that, entire blood flow circuit of the kidney is known as a Renal Circulatory System. Consequently renal
circulatory system is a subordinate system of human circulatory system. Blood come into kidney by arteries and out by veins and in a kidney no starting place or is submerged.
Mass of enter the blood = mass of outer the blood. Hence law of conservation of mass is applied for renal circulatory system. The blood flow exaggerated in presence of blood
cells. This outcome is directly relative to volume engaged by blood cells. Let X is the volume portion enclosed by the blood cells in unit volume. And X can be taken as H/100. Therefore volume portion of plasma is (1-X).
If mass ratio of blood cells to plasma is r, then we have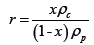 
Everywhere 
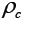and 
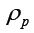are densities of blood cells and blood plasma. Actually above mass ratio is not
steady; even then it may be hypothetical to be constant in current situation. [26].
The two phase of blood, i.e., blood cells and plasma go with an ordinary velocity. In this paper we have used the model given by Campbell and Pitcher. As per this model we consider the two phases of blood separately [27]. As per the principle of conservation of mass, the equations of continuity for the two phases are as follows [28].
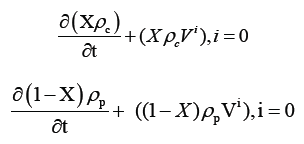
Here v is common velocity of two phases and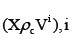 
as covariant derivative of 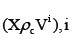with respect to Xi. As a result 
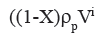with respect to Xi If we denote uniform density 
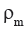by:
Equations can be collective together as follows,
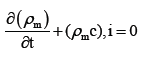
As we know that blood is incompressible liquid hence 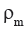will be a content measure. Hence the equation of continuity for blood flow takes the following form:
Equation of Motion: As per this principle, total momentum of every fluid system is preserved in lack of outside force. So the law of conservation of momentum is appropriate to renal circulatory system. In addition, rate of change of momentum of a fluid particle with respect to time equals to external force exerted on it, which is also known as Newton’s second
law of motion. Therefore, rate of change of momentum as same as sum of regarding two mentioned forces, which could be symbolically presented as follows.
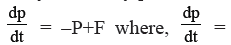rate of change of momentum,
P=Internal pressures=viscous force
The hydro dynamical pressure p among phases of blood could be hypothetical to be consistent since phases i.e. blood cells and plasma is for all time in the symmetry state in blood [29]. Consider viscosity coefficient of blood cells to be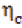 
, applying the principle of conservation of momentum, we conclude the eq. of motion for phase of blood cells:
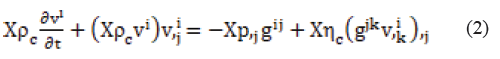
Take viscosity coefficient for plasma as . Equation of motion of plasma is:
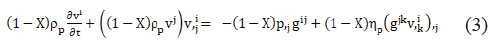
On adding up eq. (2) and (3), put in relation (1), equation of flow of blood with both phases is:
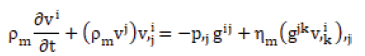
Where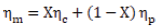 
is viscosity coefficient for blood
as a combination of two phases.
Special constitutive equations for blood: Normally blood is non-homogeneous combination of plasma and blood cells.
Although for practical reasons it can be considered to be homogeneous two-phase mixture of plasma and blood cells. The constitutive equations planned for whole blood mixture are as follows:
(i) Newtonian equation:
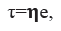
Where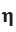 
is the viscosity coefficient
This hold fine in the broad blood vessels where there is low hematocrit [30].
(ii) The non-Newtonian power law equation:
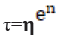
This is conformable for strain rate between 5 and 200; 0.68≤n≤ 0.80 [10]
The non -Newtonian Herschel-Bulkley equation [10]
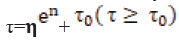
It holds superior when blood shows yield stress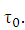 
we notice that the yield stress arise because blood cells form aggregates in the form of rouleaux at low strain rate.
If 
, no blood flow takes place. It is found that yield stress is given by the following formula
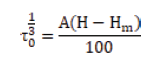
Where,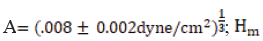 
is the hematocrit below which there is no yield stress.
Boundary Conditions: (i) The velocity of blood flow on the axis of capillaries at r=0 will be utmost and finite, say V0 = maximum velocity. (ii) The velocity of blood flow on the fence blood vessel at r=R, where, R is the radius of capillary, will be zero. This state is well known as no-slip condition.
Mathematical Modeling: Consider the two layer blood flow in that one is Newtonian while other is Non- Newtonian power law flow. The layer which is close with wall of the vessels can be taken as Newtonian; reason is layer contains plasma only. The second core layer can be considering as non-Newtonian power law, reason here the ratio of blood cells is too high in comparison to plasma.
Equation of continuity for power law flow will be:
Again the equation of the motion is extended as:
Where 
is taken from constitutive equation of power law flow
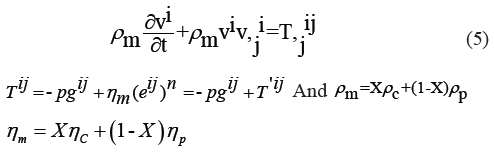
Because blood vessels are cylindrical, the above equations should transform in cylindrical co-ordinates system. Now we have to transform equation (4) and (5) in cylindrical form.
Cylindrical Co-ordinates,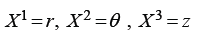 
Matrix for metric tensor as cylindrical co-ordinates:
Matrix of conjugate, metric tensor :
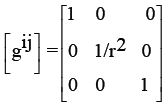
Christoffel’s symbols of 2nd kind as:
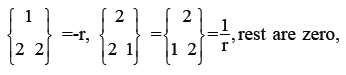
Relation among contra variant physical components of velocity of flow of blood is:
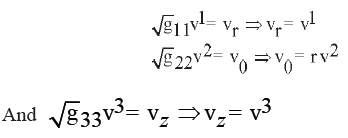
Also physical components of
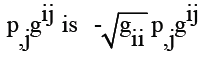
The matrix of the physical components of shearing stress-tensor:
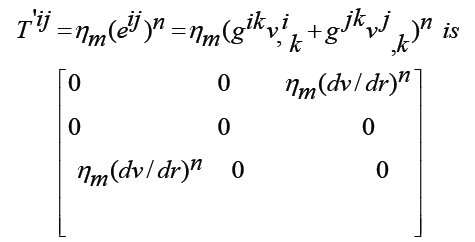
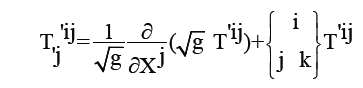
Keeping in view the above fact, the governing tensorial equation can be distorted into cylindrical form :
Equation of continuity –
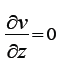
Equation of motion
R-component
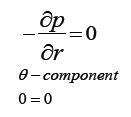
Z-component
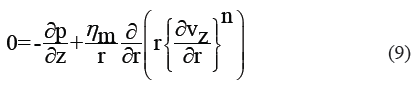
Considering flow of blood as axially symmetric in arteries
i.e. Vθ=0 and Vr
Vz and p do not depend upon θ. Also the blood flow steadily,
i.e.
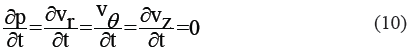
SOLUTION:
Integrating equation (6) we get, vz=v(r) because v does not depend upon θ. (11)
Integrating equation of motion (7) yields:
P=p (z) since p does not depend upon θ (12)
Now, with the help of equation (11) and (12) the equations of
motion (9) convert:
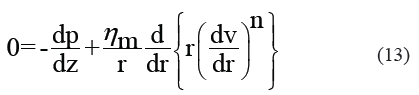
The pressure gradient (dp/dz) = p of blood flow in the arteries remote the heart which is supposed to be constant and hence the equation (13) converts:
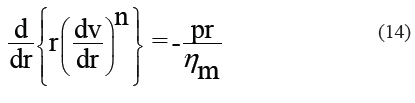
On integrating equation (14),
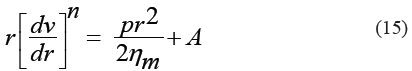
Because velocity of the blood flow on the axis of cylindrical arteries is maximum and constant. So apply the boundary condition at r=0, v=V0 (constant), equation (15) converts:
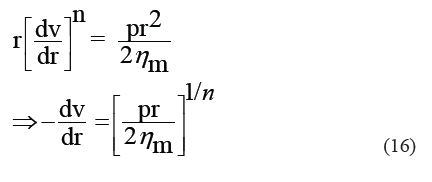
On integrating equation (15),
To determine the arbitrary constant B, apply the non-slip condition on the inner wall of the arteries at r=R, V=0, where R= radius of vessel, on equation (17),
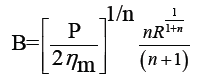
Hence the equation (17) converts:
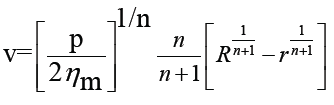
Which conclude the velocity of the blood flow in the artery remote from heart. Now the formula for velocity of blood flows can be obtained by replacing ηm with ηp in Newtonian
model:
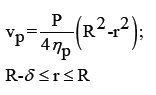
Where 
the radius of core layer. The velocity of core layer is
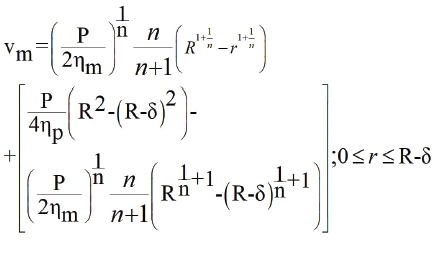
Where, the 2nd term is the relative velocity of plasma layer with respect to core layer.
Statistical Methods
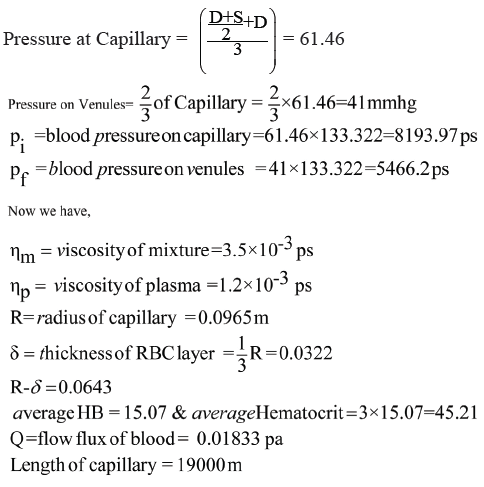
Observation data:
Bio-Physical Interpretation:
The blood flow (Flow flux) in capillary is
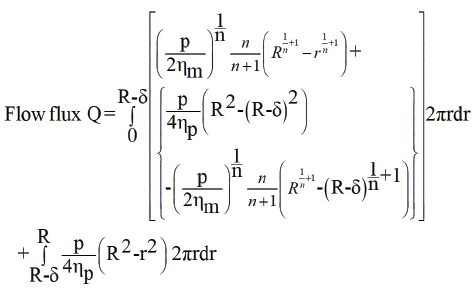
Average systolic Pressure = 125 mmhg
Average Diastolic pressure = 81.25 mmhg
References:
[1] Structure and Function of the Kidney Essay. Submitted by: ppaul11 on March 14, 2015
[2] Reddi AS.Structure and function of the kidney. In: Reddi AS.Essentials of Renal Physiology. New Jersey: College Book Publishers, 1999:21–43.
[3]. Madsen KM, Tisher CC. Anatomy of kidney. In Brenner BM, ed. Brenner and Rector’s .The kidney, 7th ed. Vol. - 1Pheladelphia: Saunders, 2004,3-72
[4] Kriz W ,Elgar M Renal anatomy .In Johnson RJ, Feehally J, eds. Comprehensive clinical nephrology ,2nd ed. Edinburgh Mosby ; 2003;1-11
[5]Cotran , RS S ; Kumar , Cotran, RS S.; Kumar, Vinay; Fausto, Nelson; Robbins, Stanley L.;Abbas, Abul K. (2005). Robbins and Cotran pathologic basis of disease. St. Louis, MO: Elsevier Saunders.ISBN 0-7216-0187-1
[6] Physiology and Anatomy. Elixir Physio. and Anatomy 89 (2015) 36723-36729
[7] Essential of Clinical Nephrology ; 1st edition ; Published by ; Dar EI Shorouk, 8 Sebawieh Al masry,Nasr City , Cairo, Egypt ; post box : 33
[8] Nutrition and Health; Nutrition Kidney disease; edited by LD Byham –Grey, JD . Burrowes and GM Chertow © Humana Press; Totowa; NJ
[9] Review of Medical physiology; 23 edition, Kim Barrett, Headwen, Brooks Scott Boitano Susan Baman ; pp-644
[10] Kapur J.N., Mathematical Models in Biology and Medicine, EWP New Delhi, 354, 1992.
[11] Shmukler, Michael (2004) “Density of the blood “The physics Factbook . Retrieved October 4 2006
[12] A.C.Guyton; Medical Physiology; Chapter 26; Urine Formation by the kidneys; I. Glomerular Filtration, Renal Blood flow and their control; PP 283-285
[13] Shmukler, Michael; Density of Blood; The Physics Factbook ; Retrieved 4 October 2006
[14]. Shoback, edited by David G Garner, Dolores (2011) “chapter 17” Greenspan’s basic and clinical endocrinology (9th Edit.). Newyork : McGraw-Hill Medical . ISBN 0-07 -162243-8
[15] Walter F. Boron (2004). Medical physiology: A cellular and Molecular Approach. Elsevier / Saunders. ISBN1-4160-2328
[16]. Sloop GD. A Unifying Theory of Atherogenesis. Med Hypothesis. 1996;47:321-5
[17] Cokelet GR, Meiselman HJ. Blood rheology. In : Baskurt OK, et al.Handbook of Hemorheology and Hemodynamics. IOS Press, 200; 45-71
[18]. ISSN: 2277-3754 ISO 9001:2008 Certified International Journal of Engineering and Innovative Technology (IJEIT) Volume 4, Issue 4, and November 2014
[19]. Sherman, I.W. and Sherman, V.G.; Biology- a human approach oxford univ. press New York, oxford; 276-277; 1989.
[20] Sapna Ratan Shah; Mathematical analysis of blood flow throw atherosclerotic arterial segment having non-symmetric and mild stenosis; International journal of research in pure and applied physics; 21 april, 2011.
[21] Purves, William K.; Sadava, David; Orians, Gordon H.; Heller, H. Craig (2004). Life: The Science of Biology (7th ed.). Sunderland, Mass: Sinauer Associates. p. 954. ISBN 0-7167-98565.
[22] Stuart J, Kenny MW: Blood rheology. J din Pathol 19803:417-429
[23] Chien S: Blood rheology in hypertension and cardiovascular disease. Cardiovasc Med 1977; 2:356-360
[24] Berkow, Robert, ed. Merck Manual of Medical Information. Whitehouse Station, NJ: Merck Research Laboratories, 1997
[25] Fogelson, A.L.; A mathematical model and numerical method for studying platelet adhesion and aggregation during blood clotting; J.comput. Physics; 56; 1984.
[26] Singh P. and Upadhyay K.S. ; A New approach for the shock propagation in two phases system; Nat.Acad.Sci.Letters, Vol 8, No 2, 1985
[27] Compbell, I.J. and Pitcher, A.S.; Shock waves in a liquid containing gas bubbles, proc.Roy.Soc; A 243, 1958. [28] Kapur,J.N; and Gupta, R.c ; Power-law fluid flow in the inlet length of a circular pipe; The math, seminar; 3, 55-67; 1963.
[29] Ruch, T.C. and H.D., Patton, (Eds); Physiology and Biophysics-volsii and iii, W.B.S.; 1973
[30] Taylor, M.G.; Hemodynamics. Ann.Rev. Physiol; 35; 87, 1973
|
IJCRR 
This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License
